

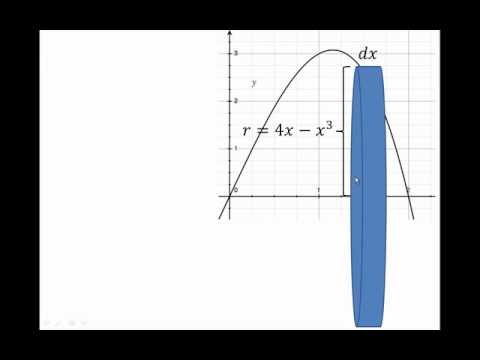
If you're unable to graph the given equations, to see the region being rotated, or you're not sure how to draw one representative "slice" whose rotation forms a single shell (or washer), let us know. You can then use the diagram as a guide for determining what gets subtracted from what and where the boundaries lie. That is, draw a sample of one rectangular region that will be rotated around the y-axis. The other method uses shells (sort of like wrapping outer tubes around inner tubes, to build up the solid.)Įither way (washers or shells), draw a representative slice, on your graph. The revolving regions can be in the XY plane on a vertical line in the y-axis or it can be on the horizontal. By making slight modifications to these methods, we can find volumes of solids of revolution resulting from revolving regions. Have you already decided on a method? In your subject line, you wrote "Disk or Washer", but those are two names for the same method (washers are disks, with a hole in the center, and integration stacks them, to form the solid). The washer method and the shell method are powerful methods for finding the volumes of solids of revolution. With shells, you can use the functions as given, integrating with respect to dx, but you'll need to sum a pair of integrals. With washers, you would integrate with respect to dy, and that would require solving y=5x for x because that x is the inner radius of each washer. (It's an upside down cone, with a smaller, cone-shaped hollow space in its center.) Knowing the general shape, you can think about what the individual pieces look like.įor these pieces, you have a choice between using washers or shells. This helps to visualize the shape of the object. Have you graphed the region to be rotated, on the xy-plane? It's in Quadrant I. Click to expand.What have you done, so far, thinking about setting up the integral(s)?


 0 kommentar(er)
0 kommentar(er)
